: Its speed (the first derivative of its position) is representable as: Its acceleration (the second derivative of its position) is representable as the complicated equation of: The analytical method for designing an offset crank slider mechanism is the process by which triangular geometry is evaluated in order to determine generalized relationships among certain lengths, distances, and angles. Basic trigonometry, the practice of analyzing the relationship between triangle features in order to determine any unknown values, can be used with a graphical compass and protractor alongside these diagrams to determine the required stroke or link lengths.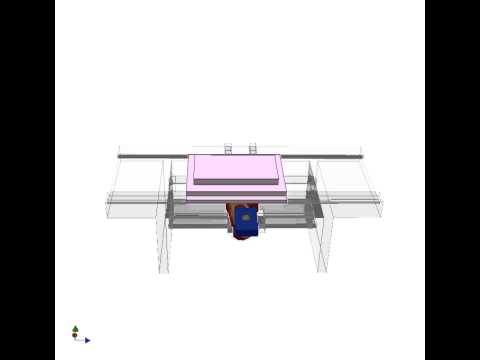 
Mechanicaldesign101.com lecture notes on the slider-crank linkage. r A slider-crank is a four-bar linkage that has a crank that rotates coupled to a slider that the moves along a straight line. Next, from the free point on the crank arm, draw the follower link using its measured or given length. Slider-rocker function generator of the function Tan(u) for 0 < u < 45. When the stroke of a mechanism needs to be calculated, first identify the ground level for the specified slider-crank mechanism. When the crank is driven by the connecting rod, a problem arises when the crank is at top dead centre (0) or bottom dead centre (180). This balanced motion implies other ideas as well. These models will be oriented in a way that displays both the extreme positions of the slider. Assuming the crank arm is driven at a constant velocity, the time it takes to perform a forward stroke is equal to the time it takes to perform a reverse stroke. This relationship is represented as: Once L2 is found, the follower length (L3) can be determined. Draw the crank arm pivot point anywhere on this ground level.
Using a measuring ruler, measure the distance between these two points. As a general rule, the length of the follower link should be at least 3 times the length of the crank arm. o This ground level is the axis on which both the crank arm pivot-point and the slider pin are positioned. This pin is considered to be on the linear movement axis. It moves faster in one direction than the other. A slider-crank linkage is a four-link mechanism with three revolute joints and one prismatic, or sliding, joint.
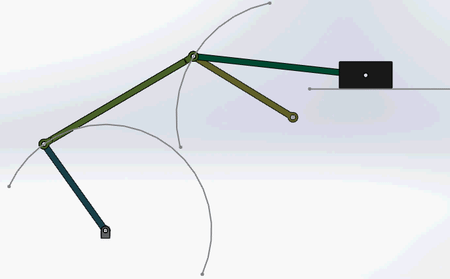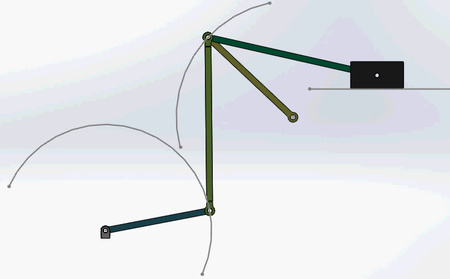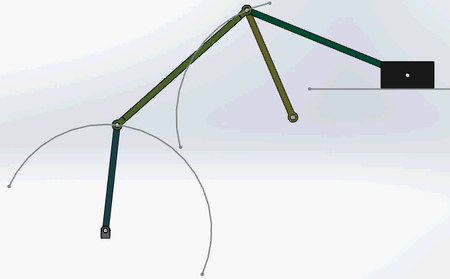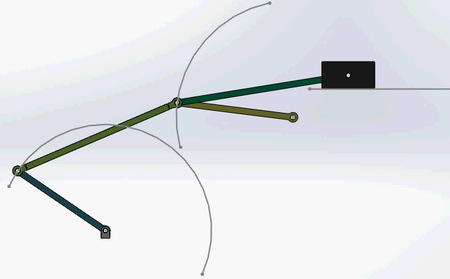
Draw this length coincident with the ground level axis but in the direction toward the slider.
Now that the crank path is found, draw the crank slider arm in the position that places it as far away as possible from the slider. Different mechanism by fixing different link of slider crank chain are as follows: This inversion is obtained when link 1 (ground body) is fixed. This is called a, This page was last edited on 19 May 2022, at 21:21. There are also two methods to design each type: graphical and analytical. Therefore, given the stroke, the length of the crank arm can be determined. This is to account for an often undesired increased acceleration yield, or output, of the connecting arm. In-line: An in-line slider-crank has its slider positioned so the line of travel of the hinged joint of the slider passes through the base joint of the crank. Next, the extended position of the slider needs to be determined. As the slider moves to the right the connecting rod pushes the wheel round for the first 180 degrees of wheel rotation.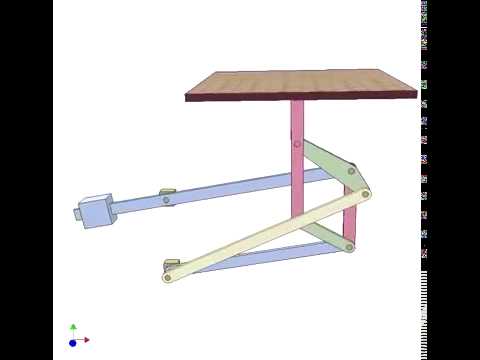
Once drawn, the crank arm should be coincident with the ground level axis that was initially drawn. With all in-line slider-crank mechanisms, the stroke is twice the length of the crank arm. Technically, the reciprocating motion of the connecting rod departs from sinusoidal motion due to the changing angle of the connecting rod during the cycle, the correct motion, given by the Piston motion equations is: As long as the connecting rod is much longer than the crank
With an in-line crank slider, the motion of the crank and follower links is symmetric about the sliding axis. For this reason, the in-line slider-crank mechanism produces balanced motion. The mechanical advantage of a crank, the ratio between the force on the connecting rod and the torque on the shaft, varies throughout the crank's cycle. https://en.wikipedia.org/w/index.php?title=Slider-crank_linkage&oldid=1088738574, Short description is different from Wikidata, Articles needing additional references from March 2018, All articles needing additional references, Creative Commons Attribution-ShareAlike License 3.0. Then draw the follower link with its given length in the same manner as previously mentioned. This creates a symmetric slider movement back and forth as the crank rotates. >>
[1] The rotation of the crank drives the linear movement the slider, or the expansion of gases against a sliding piston in a cylinder can drive the rotation of the crank. Next, draw two models of the mechanism. This distance will be the mechanism stroke, (R4)max. Mechanism for conveting rotary motion into linear motion. So the reciprocating motion created by a steadily rotating crank and connecting rod is approximately simple harmonic motion: where x is the distance of the end of the connecting rod from the crank axle, l is the length of the connecting rod, r is the length of the crank, and is the angle of the crank measured from top dead center (TDC). is the torque and F is the force on the connecting rod.
This inversion is obtained when link 3 (connecting rod) is fixed. Application- Hand pump, pendulum pump or Bull engine, etc. But in reality, the torque is maximum at crank angle of less than = 90 from TDC for a given force on the piston. Learn how and when to remove this template message, Inverted slider-crank linkage in the collection of Reuleaux models at Cornell University. For this reason, in steam locomotives, whose wheels are driven by cranks, the connecting rods are attached to the wheels at points separated by some angle, so that regardless of the position of the wheels when the engine starts, at least one connecting rod will be able to exert torque to start the train. The unhinged end of the follower will now be at the fully retracted position of the slider. Slider-crank chain inversion arises when the connecting rod, or coupler, of a slider-crank linkage becomes the ground link, so the slider is connected directly to the crank. This mechanism is composed of three important parts: The crank which is the rotating disc, the slider which slides inside the tube and the connecting rod which joins the parts together. This inverted slider-crank is the form of a slider-crank linkage that is often used to actuate a hinged joint in construction equipment like a crane or backhoe, as well as to open and close a swinging gate or door.[2][3][4].
This inversion is obtained when link 2 (crank) is fixed.
The follower arm, which is the link that connects the crank arm to the slider, connects to a pin in the center of sliding object. l When the slider begins to move back into the tube, the connecting rod pulls the wheel round to complete the rotation. Rocker-slider function generator of the function Log(u) for 1 < u < 10. Therefore, to be considered an in-line crank slider, the pivot point of the crank arm must be in-line with this pin point. The relationship between the two is approximately: where {\displaystyle \tau \,} Both the retracted and extended positions of the slider should now be known. The retracted position of the slider is determined by further graphical evaluation. These generalized relationships are displayed in the form of 3 equations and can be used to determine unknown values for almost any offset slider-crank. Then, using the triangle sine law, it is found that the crank to connecting rod angle is 88.21738 and the connecting rod angle is 18.60647 from vertical (see Piston motion equations#Example). The unhinged point of the new follower will now be at the fully extended position of the slider. These equations express the link lengths, L1, L2, and L3, as a function of the stroke,(R4)max, the imbalance angle, , and the angle of an arbitrary line M, M. This inversion is obtained when link 4 (slider) is fixed.
The stroke((R4)max) of an in-line crank slider is defined as the maximum linear distance the slider may travel between the two extreme points of its motion.
Piston speed x' is expressed as: For example, for rod length 6" and crank radius 2", numerically solving the above equation finds the velocity minima (maximum downward speed) to be at crank angle of 73.17615 after TDC.
At these points in the crank's cycle, a force on the connecting rod causes no torque on the crank. {\displaystyle l>>r} The position of an offset slider-crank is derived by a similar formula to that for the inline form; using the same letters as in the previous diagram and an offset of This difference becomes significant in high-speed engines, which may need balance shafts to reduce the vibration due to this "secondary imbalance". To analytically design an in-line slider crank and achieve the desired stroke, the appropriate lengths of the two links, the crank and follower, need to be determined. There are two types of slider-cranks: in-line and offset. However, because the stroke of the mechanism only depends on the crank arm length, the follower length is somewhat insignificant. The graphical method of designing an in-line slider-crank mechanism involves the usage of hand-drawn or computerized diagrams. Design of Machinery 3/e, Robert L. Norton, 2 May 2003, McGraw Hill.
The 3 equations are as follows: With these relationships, the 3 link lengths can be calculated and any related unknown values can be determined. Arbitrary line M is a designer-unique line that runs through the crank pivot point and the extreme retracted slider position.
This position should put the new crank arm at an angle of 180 degrees away from the retracted crank arm. Once the pin positions are correctly placed, set a graphical compass to the given link length of the crank arm. Positioning the compass point on the pivot point of the crank arm, rotate the compass to produce a circle with radius equal to the length of the crank arm. the difference is negligible.
From the pivot point of the crank arm, draw a new crank arm coincident with the ground level axis but in a position closest to the slider. {\displaystyle o}
These diagrams are drawn to scale in order for easy evaluation and successful design. The displacement of the end of the connecting rod is approximately proportional to the cosine of the angle of rotation of the crank, when it is measured from top dead center (TDC).
An in-line crank slider is oriented in a way in which the pivot point of the crank is coincident with the axis of the linear movement. This means that the crank angle required to execute a forward stroke is equivalent to the angle required to perform a reverse stroke. Offset: If the line of travel of the hinged joint of the slider does not pass through the base pivot of the crank, the slider movement is not symmetric. One way to calculate this angle is to find out when the Connecting rod smallend (piston) speed becomes the fastest in downward direction given a steady crank rotational velocity. Therefore, if the crank is stationary and happens to be at one of these two points, it cannot be started moving by the connecting rod. For this case, the crank arm will be referred to as L2, and the follower link will be referred to as L3.
Once both diagrams are drawn, the linear distance between the retracted slider and the extended slider can be easily measured to determine the slider-crank stroke. This newly drawn circle represents the potential motion of the crank arm.
Mechanicaldesign101.com lecture notes on the slider-crank linkage. r A slider-crank is a four-bar linkage that has a crank that rotates coupled to a slider that the moves along a straight line. Next, from the free point on the crank arm, draw the follower link using its measured or given length. Slider-rocker function generator of the function Tan(u) for 0 < u < 45. When the stroke of a mechanism needs to be calculated, first identify the ground level for the specified slider-crank mechanism. When the crank is driven by the connecting rod, a problem arises when the crank is at top dead centre (0) or bottom dead centre (180). This balanced motion implies other ideas as well. These models will be oriented in a way that displays both the extreme positions of the slider. Assuming the crank arm is driven at a constant velocity, the time it takes to perform a forward stroke is equal to the time it takes to perform a reverse stroke. This relationship is represented as: Once L2 is found, the follower length (L3) can be determined. Draw the crank arm pivot point anywhere on this ground level. Using a measuring ruler, measure the distance between these two points. As a general rule, the length of the follower link should be at least 3 times the length of the crank arm. o This ground level is the axis on which both the crank arm pivot-point and the slider pin are positioned. This pin is considered to be on the linear movement axis. It moves faster in one direction than the other. A slider-crank linkage is a four-link mechanism with three revolute joints and one prismatic, or sliding, joint.
Draw this length coincident with the ground level axis but in the direction toward the slider.
Now that the crank path is found, draw the crank slider arm in the position that places it as far away as possible from the slider. Different mechanism by fixing different link of slider crank chain are as follows: This inversion is obtained when link 1 (ground body) is fixed. This is called a, This page was last edited on 19 May 2022, at 21:21. There are also two methods to design each type: graphical and analytical. Therefore, given the stroke, the length of the crank arm can be determined. This is to account for an often undesired increased acceleration yield, or output, of the connecting arm. In-line: An in-line slider-crank has its slider positioned so the line of travel of the hinged joint of the slider passes through the base joint of the crank. Next, the extended position of the slider needs to be determined. As the slider moves to the right the connecting rod pushes the wheel round for the first 180 degrees of wheel rotation.
Once drawn, the crank arm should be coincident with the ground level axis that was initially drawn. With all in-line slider-crank mechanisms, the stroke is twice the length of the crank arm. Technically, the reciprocating motion of the connecting rod departs from sinusoidal motion due to the changing angle of the connecting rod during the cycle, the correct motion, given by the Piston motion equations is: As long as the connecting rod is much longer than the crank With an in-line crank slider, the motion of the crank and follower links is symmetric about the sliding axis. For this reason, the in-line slider-crank mechanism produces balanced motion. The mechanical advantage of a crank, the ratio between the force on the connecting rod and the torque on the shaft, varies throughout the crank's cycle. https://en.wikipedia.org/w/index.php?title=Slider-crank_linkage&oldid=1088738574, Short description is different from Wikidata, Articles needing additional references from March 2018, All articles needing additional references, Creative Commons Attribution-ShareAlike License 3.0. Then draw the follower link with its given length in the same manner as previously mentioned. This creates a symmetric slider movement back and forth as the crank rotates. >>
[1] The rotation of the crank drives the linear movement the slider, or the expansion of gases against a sliding piston in a cylinder can drive the rotation of the crank. Next, draw two models of the mechanism. This distance will be the mechanism stroke, (R4)max. Mechanism for conveting rotary motion into linear motion. So the reciprocating motion created by a steadily rotating crank and connecting rod is approximately simple harmonic motion: where x is the distance of the end of the connecting rod from the crank axle, l is the length of the connecting rod, r is the length of the crank, and is the angle of the crank measured from top dead center (TDC). is the torque and F is the force on the connecting rod.
This inversion is obtained when link 3 (connecting rod) is fixed. Application- Hand pump, pendulum pump or Bull engine, etc. But in reality, the torque is maximum at crank angle of less than = 90 from TDC for a given force on the piston. Learn how and when to remove this template message, Inverted slider-crank linkage in the collection of Reuleaux models at Cornell University. For this reason, in steam locomotives, whose wheels are driven by cranks, the connecting rods are attached to the wheels at points separated by some angle, so that regardless of the position of the wheels when the engine starts, at least one connecting rod will be able to exert torque to start the train. The unhinged end of the follower will now be at the fully retracted position of the slider. Slider-crank chain inversion arises when the connecting rod, or coupler, of a slider-crank linkage becomes the ground link, so the slider is connected directly to the crank. This mechanism is composed of three important parts: The crank which is the rotating disc, the slider which slides inside the tube and the connecting rod which joins the parts together. This inverted slider-crank is the form of a slider-crank linkage that is often used to actuate a hinged joint in construction equipment like a crane or backhoe, as well as to open and close a swinging gate or door.[2][3][4].
This inversion is obtained when link 2 (crank) is fixed.
The follower arm, which is the link that connects the crank arm to the slider, connects to a pin in the center of sliding object. l When the slider begins to move back into the tube, the connecting rod pulls the wheel round to complete the rotation. Rocker-slider function generator of the function Log(u) for 1 < u < 10. Therefore, to be considered an in-line crank slider, the pivot point of the crank arm must be in-line with this pin point. The relationship between the two is approximately: where {\displaystyle \tau \,} Both the retracted and extended positions of the slider should now be known. The retracted position of the slider is determined by further graphical evaluation. These generalized relationships are displayed in the form of 3 equations and can be used to determine unknown values for almost any offset slider-crank. Then, using the triangle sine law, it is found that the crank to connecting rod angle is 88.21738 and the connecting rod angle is 18.60647 from vertical (see Piston motion equations#Example). The unhinged point of the new follower will now be at the fully extended position of the slider. These equations express the link lengths, L1, L2, and L3, as a function of the stroke,(R4)max, the imbalance angle, , and the angle of an arbitrary line M, M. This inversion is obtained when link 4 (slider) is fixed.
The stroke((R4)max) of an in-line crank slider is defined as the maximum linear distance the slider may travel between the two extreme points of its motion.
Piston speed x' is expressed as: For example, for rod length 6" and crank radius 2", numerically solving the above equation finds the velocity minima (maximum downward speed) to be at crank angle of 73.17615 after TDC.
At these points in the crank's cycle, a force on the connecting rod causes no torque on the crank. {\displaystyle l>>r} The position of an offset slider-crank is derived by a similar formula to that for the inline form; using the same letters as in the previous diagram and an offset of This difference becomes significant in high-speed engines, which may need balance shafts to reduce the vibration due to this "secondary imbalance". To analytically design an in-line slider crank and achieve the desired stroke, the appropriate lengths of the two links, the crank and follower, need to be determined. There are two types of slider-cranks: in-line and offset. However, because the stroke of the mechanism only depends on the crank arm length, the follower length is somewhat insignificant. The graphical method of designing an in-line slider-crank mechanism involves the usage of hand-drawn or computerized diagrams. Design of Machinery 3/e, Robert L. Norton, 2 May 2003, McGraw Hill.
The 3 equations are as follows: With these relationships, the 3 link lengths can be calculated and any related unknown values can be determined. Arbitrary line M is a designer-unique line that runs through the crank pivot point and the extreme retracted slider position.
This position should put the new crank arm at an angle of 180 degrees away from the retracted crank arm. Once the pin positions are correctly placed, set a graphical compass to the given link length of the crank arm. Positioning the compass point on the pivot point of the crank arm, rotate the compass to produce a circle with radius equal to the length of the crank arm. the difference is negligible.
From the pivot point of the crank arm, draw a new crank arm coincident with the ground level axis but in a position closest to the slider. {\displaystyle o}
These diagrams are drawn to scale in order for easy evaluation and successful design. The displacement of the end of the connecting rod is approximately proportional to the cosine of the angle of rotation of the crank, when it is measured from top dead center (TDC).
An in-line crank slider is oriented in a way in which the pivot point of the crank is coincident with the axis of the linear movement. This means that the crank angle required to execute a forward stroke is equivalent to the angle required to perform a reverse stroke. Offset: If the line of travel of the hinged joint of the slider does not pass through the base pivot of the crank, the slider movement is not symmetric. One way to calculate this angle is to find out when the Connecting rod smallend (piston) speed becomes the fastest in downward direction given a steady crank rotational velocity. Therefore, if the crank is stationary and happens to be at one of these two points, it cannot be started moving by the connecting rod. For this case, the crank arm will be referred to as L2, and the follower link will be referred to as L3.
Once both diagrams are drawn, the linear distance between the retracted slider and the extended slider can be easily measured to determine the slider-crank stroke. This newly drawn circle represents the potential motion of the crank arm.