x , & = -0.5 \\ {\displaystyle \mathbb {R} \setminus (-1,1)=(-\infty ,-1]\cup [1,\infty )} {\displaystyle z} This is the same as before but we have to remember the period of the graph to list the rest of the solutions. = respectively. etc. We must always consider other possibilities when using inverse trigonometric functions. They say this in order to guarantee you will hire them in your time of need. {\displaystyle \,\iff \,} = c = ( since tangent is nonnegative on To use this website, please enable javascript in your browser. {\displaystyle \int u\,dv=uv-\int v\,du} y {\displaystyle b} x {\displaystyle \theta } {\displaystyle \,\iff \,} v ( k Use these tips to find a great locksmith ahead of time. Nevertheless, certain authors advise against using it for its ambiguity. any whole number, both positive and negative). {\displaystyle \operatorname {arccsc}(x)=\arcsin(1/x)} arctan 2 i sec {\displaystyle \cos \theta =x.} {\displaystyle y} = Thus, the principal value of tan-13 is /3. The further the metal piece is pushed into the whole, the longer it will take to remove it. There exists a unique value '' in [0, ] satisfying cos = k for k ∈ [ 1, 1]. R , 2 0 0 < If In the first quadrant we get only we get positive valuesfor all trigonometric ratios.So we have to choose one of the angles from 0 to-/2 that is negative angle. Cada servicio es pensado desde sus inicios con el objetivo de respetar y potenciar la imagen de marca. ( : Recall that in a circle of radius \(r\), the arc length subtended by an angle \(\theta\) radians has length \(r\,\theta\). 2 By setting one of the three sides equal to 1 and one of the remaining sides equal to our input sin They could be running a scam. = The principal value of satisfying the equation
x {\displaystyle y={\sqrt {x}}} x But we need to be careful because these functions are are not one-to-one; in fact they are periodic, so for instance there are infinitely many values of \(x\) that give the same value of \(\sin x\). 2. But the 0 is known as principal value. For example, using function in the sense of multivalued functions, just as the square root function ( cos x 2 has one solution in this interval called the principal value of . 2 {\displaystyle \pi n} Organizacin integral del evento, conduccin, video y pantallas gigantes, sonido y musicalizacin, personal promocional, ambientacin de Saln VIP 1er piso, ambientacin de boxes de exhibicin y saln VIP en boxes, show de drift y show para la develacin de la coup, cobertura del evento en VIP y en pista con transmisin en vivo, cmaras a bordo de vehculos de prueba, uniformes promocionales y buzos antiflama de los pilotos, cascos. rounds to the nearest integer. z The problem with that is they can do a shabby job or overcharge you. The process of finding the solution set is called solving the trigonometric equation. Well use \(\arctan\), \(\arccos\) and \(\arcsin\) in this resource. 2 As you know by now, the values of 'sin x' and 'cos x' repeat after an interval of 2 rad (or 360) whereas the values of 'tan x' repeat after an interval of (or 180). ( = The principal value of cos (3/2) is /6 as /6 \[[0,\pi ]\]. {\displaystyle \sin \theta =\pm 1,} = ( The Minkowski sum notation for some {\displaystyle \sec \theta =-1} that is used above to concisely write the domains of Hence, since 2009, the ISO 80000-2 standard has specified solely the "arc" prefix for the inverse functions. b) sec = k, k ∈ ( , 1] [1, ) lies in [0, ] {}
{\displaystyle k\in \mathbb {Z} .} [6][16] Another precarious convention used by a tiny number of authors is to use an uppercase first letter, along with a 1 superscript: Sin1(x), Cos1(x), Tan1(x), etc. = ( {\displaystyle \cos \theta =-1} w and 1 2 2 k {\displaystyle \theta .} ) {\displaystyle y=\arcsin(x)} Fields marked with an asterisk (*) are required. Some of the issues that arise for an inverse of \(\tan x\) also arise for \(\sin x\) and \(\cos x\), but well see that we have to be even more careful for these functions. As the principal value of tan-1 is (/2, /2). One possible way of defining the extension is: where the part of the imaginary axis which does not lie strictly between the branch points (i and +i) is the branch cut between the principal sheet and other sheets. For example, suppose a roof drops 8 feet as it runs out 20 feet. = For z on a branch cut, the path must approach from Re[x] > 0 for the upper branch cut and from Re[x] < 0 for the lower branch cut. 2 yields the final result: Since the inverse trigonometric functions are analytic functions, they can be extended from the real line to the complex plane. In this sense, all of the inverse trig functions can be thought of as specific cases of the complex-valued log function. 2 e {\displaystyle \theta } cos With the help of the best study materials, students can easily prepare the Chapter "Inverse Trigonometric Functions" for their Maths examination. Plan de lanzamiento de productos mediante actividades tcticas de comunicacin, BTL below the line y publicidad. The second solution, (or secondary value), is found using the fact that tan is also positive in the third quadrant (it repeats every radians). whereas with the range 2 = {\displaystyle x=0} Because all of the inverse trigonometric functions output an angle of a right triangle, they can be generalized by using Euler's formula to form a right triangle in the complex plane. . + However, this might appear to conflict logically with the common semantics for expressions such as sin2(x) (although only sin2 x, without parentheses, is the really common one), which refer to numeric power rather than function composition, and therefore may result in confusion between multiplicative inverse or reciprocal and compositional inverse. General Solutions of Trigonometric Equations. ), Another series for the arctangent function is given by, where arccsc = sin = x LHS) and right hand side (i.e. {\displaystyle \cos \theta =-1} ) x 
sin \sin {\pi \over 6} & = {1 \over 2} \\ sin Desarmable para poder trasladarlo en un semirremolque. La concebimos de forma integral cuidndola y maximizando su eficacia en todos sus aspecto. {\displaystyle \,\pm ,\,} sin You are sure to be happy that you did. 1 It is the value of \(x\) in the interval \(\left(-\tfrac{\pi}{2},\tfrac{\pi}{2}\right)\) such that \(\tan x=\sqrt{3}\). Therefore, (3 + 45) = 120 360n and (3 + 45) = 120 360n. Along with the notes, they will also discover the sample papers and important questions. This means that when solving trigonometric equations with a multiple of , there will be a different number of solutions in a 3600 range. Its inverse is \(\arcsin x\) which has domain \([-1,1]\). {\displaystyle y} We have to think about the angle of cos for which we get the value3/2. ( x ( {\displaystyle x,} cos {\displaystyle \csc } There are two methods to find the solution of a trigonometric equation: Use the graph of the trigonometric functions. 3 1 ) = For a given real number ) is denoted by, The symbol i.e. ln 2 < 1 < k and We generally use Cosecant or csc to determine the reciprocal of sin. it is not possible for the LHS statement to be true and also simultaneously for the RHS statement to false), because otherwise "LHS , For z not on a branch cut, a straight line path from 0 to z is such a path. 1 The arcsine function may then be defined as: where (the square-root function has its cut along the negative real axis and) the part of the real axis which does not lie strictly between 1 and +1 is the branch cut between the principal sheet of arcsin and other sheets; where the part of the real axis between 1 and +1 inclusive is the cut between the principal sheet of arcsec and other sheets; These functions may also be expressed using complex logarithms. (2 sin 1) (sin + 1) = 0, where 0 360. sin = 0.5 or sin = -1 and solve as before to get, = 30, 150, or 270. ) Diseo de banderas y carteles para tribunas y accesos. sec Diseo grfico, industrial, audiovsual aplicado a las necesidades comunicacionales y funcionales del cliente. {\displaystyle \,-\pi \leq \theta \leq \pi \,} arccos x , {\displaystyle n,}, Domain of tangent x is a function that is decreasing in two different intervals cosec, Some Significant Properties Of Inverse Trigonometric Functions, (cosec ) = /2 , [/2, 0][0, /2]. {\textstyle 0 x is an odd function that falls in the range of /2, /2. Recalling the right-triangle definitions of sine and cosine, it follows that. ) Solutions to elementary trigonometric equations, Relationships between trigonometric functions and inverse trigonometric functions, Relationships among the inverse trigonometric functions, Derivatives of inverse trigonometric functions, Indefinite integrals of inverse trigonometric functions, Arctangent function with location parameter, To clarify, suppose that it is written "LHS, Differentiation of trigonometric functions, List of integrals of inverse trigonometric functions, "Chapter II. then applying the Pythagorean theorem and definitions of the trigonometric ratios. and it will be odd if } It is the inverse of the tangent function. ) to be used; that solution being: These can be further simplified using the logarithmic definitions of the inverse hyperbolic functions: The absolute value in the argument of the arcosh function creates a negative half of its graph, making it identical to the signum logarithmic function shown above. 0 Idea creativa y diseo de campaa publicitaria. {\displaystyle i={\sqrt {-1}}} exactly one of these two equalities can hold. \therefore \sin^{-1} (-0.5) & = -{\pi \over 6} (for fixed is the length of the hypotenuse. n ) = ) or This page will be removed in future. For any integer n, 2n + is also a solution of given trigonometric equation. Z Solve the equation cos = 0, for +. {\displaystyle \theta } {\displaystyle \arcsin(x)} {\displaystyle \theta =\pi +2\pi k=-\pi +2\pi (1+k)} {\displaystyle (-1,1).}. {\textstyle \sin \left({\frac {\pi }{2}}-\theta \right)=\cos \theta ,} We can use the symmetry and periodicity of the \(\tan x\) graph to express all solutions of \(\tan x=\sqrt{3}\) in terms of \(\arctan \sqrt{3}\). , is complex-valued, we have to use the final equation so that the real part of the result isn't excluded. {\displaystyle \theta } , , r The table below shows the values of a, b, and c for each of the inverse trig functions and the equivalent expressions for 1 sec b y {\displaystyle \mathbb {R} } and Find the principal solution of the following equations. ) ( ( {\displaystyle \cos } = The graph of the function sin c where c is a constant, is a sine wave with a period of 2 c. The frequency is c times that of sin . For example.. We know that the principal value of the trigonometric function at a point is the value of the inverse function at a point , which falls in the range of the principal values unit. = 2 (that is, real numbers) that are not in the interval c Armado de un sector VIP junto al palenque, ambientacin, mobiliario, cobertura del evento, servicio de catering. cos (where We also need the range of the restricted function to be the same as the range of the original function. Apsis, creada en 2004, cuenta con un equipo de diseadores grficos, industriales, arquitectos, ingenieros, licenciados en letras especializados en publicidad y profesionales independientes provenientes de diferentes reas pero aunados todos en un objetivo comn: la comunicacin exitosa del cliente. Since the length of the hypotenuse doesn't change the angle, ignoring the real part of cosec-1 x is an odd function that falls in the range of /2, /2. Since cos x is positive the principal value is in the first quadrant. In these situations find the two initial solutions, make the general set of solutions, and then rearrange to find . We are given Inverse Trigonometric Functions in Maths is simply defined as the inverse of some basic trigonometric functions such as sine, cosine, tan, sec, cosec and cot. (See if you can get these solutions. , {\displaystyle s,} x The inverse of the cos function is called arccosine. ) + Dont be one of them. Use the four quadrants of the coordinate grid. and 1 b = Note: Some authors[citation needed] define the range of arcsecant to be sin {\displaystyle \mathbb {Z} =\{\ldots ,\,-2,\,-1,\,0,\,1,\,2,\,\ldots \}} RHS" indicates that either (a) the left hand side (i.e. {\displaystyle \,\iff \,} can be transformed into It implies inverse trigonometric functions are beneficial whenever the sides of a triangle are known and we want to determine the angles of a triangle. All of the three functions periodically repeat their values and the simplest way to learn this, is to make sure that you understand the general rules below which use 'n' to represent any integer (i.e. is defined so that Sec-1 x is neither an odd nor an even function. x Any equation for sin = S for the domain. v k They are the set of all angles 1 involve the "plus or minus" symbol Z Nuestra filosofa de trabajo es apostar siempre al compromiso, como un camino ineludible para lograr los objetivos de nuestros clientes. [11] In computer programming languages, the inverse trigonometric functions are often called by the abbreviated forms asin, acos, atan.[12]. We have to think about the angle of cos for which we get the value, [0, ]. In principle, inverse trigonometric functions should be functions that undo the effects of functions such as \(\sin x\), \(\cos x\) and \(\tan x\). Diseo y programacin de aplicaciones interactivas para eventos. Diseo y construccin de maqueta en acrlico con el sistema Anti Jamming funcionando. The cosine function from [0, ] → [ 1, 1] is bijective. Simply taking the imaginary part works for any real-valued From here, we can solve for For angles near 0 and , arccosine is ill-conditioned and will thus calculate the angle with reduced accuracy in a computer implementation (due to the limited number of digits). 1 Locksmith Advice That You Should Not Miss, The Best Locksmith Tips To Handle Your Locks Yourself, Exploring Systems In Locksmith Home Security. Click. For example the value of cos o is 1, the value of cos 2, 4 , are also 1. {\displaystyle \cos \theta =x} 1 R Others will tack on a fee if they have to drive a certain distance. . They are the set of all angles Denoted by \[tan^{-1} x\]. Whenever we have cos the principal value ofmust be in[0, ]. sin {\displaystyle \varphi } 2 cos and We have to choose one of the angles fromthe first or second quadrant. {\displaystyle \tan(\operatorname {arcsec}(x))={\sqrt {x^{2}-1}},} in the domain, the expression All rights reserved. If they do not provide one, ask them for it. 1 , To avoid this confusion, some people choose to represent the inverse function by using arc as a prefix. Hence, the value of cos-1 (1/2) + 2 sin-1 (1/2) is 2 /3. csc If the trigonometric equations are satisfied by all values of unknown angles, for which the functions are defined, then they are called identities. 1. The maximum value of sec-1 x is , and it lies at x = 1, The minimum value of sec-1 x is -, and it lies at x = -1, Sec-1 x is a function that is decreasing in two different intervals, Let y = cosec-1 x, |x| 1, y (/2,0) ( 0,/2). = {\displaystyle \tan \left({\tfrac {\theta }{2}}\right)={\tfrac {\sin(\theta )}{1+\cos(\theta )}}} This notation arises from the following geometric relationships:[citation needed] Several notations for the inverse trigonometric functions exist. sin x when measuring in radians, an angle of radians will correspond to an arc whose length is r, where r is the radius of the circle. {\displaystyle \cot ,\csc ,\tan ,{\text{ and }}\sec } ) , because the tangent function is nonnegative on this domain. for some {\displaystyle \tan(\operatorname {arcsec}(x))=\pm {\sqrt {x^{2}-1}},} This extends their domains to the complex plane in a natural fashion. are the same. , {\displaystyle \varphi =(-1)^{k}\arcsin(x)+\pi k\;{\text{ for some }}k\in \mathbb {Z} ,} \sin {\pi \over 6} & = 0.5 \\ ] The following graphs shows how we could restrict the domain of \(\tan x\) and the resulting function \(\arctan x\). {\displaystyle \operatorname {rni} } {\displaystyle a^{2}+b^{2}=h^{2}} The following table shows how inverse trigonometric functions may be used to solve equalities involving the six standard trigonometric functions. ) In other words, the solutions of a trigonometric equation for which 'x' lies in the interval 0 to 2, i.e., 0 x 2, are called the principal solution. = {\displaystyle \theta } is the adjacent side,
Arccosecant - This function is the inverse of the cosecant function and is denoted by \[cosec^{-1} x\]. tan {\displaystyle \sec \theta =-1} Y trabajamos junto a ellos en busca de los mejores resultados a travs de mensajes y estrategias contundentes que posicionen a la marca en un mercado cada da ms competitivo. 2 The series for arctangent can similarly be derived by expanding its derivative But principal value must be in[-/2,/2]. {\displaystyle b} R u Only the solution to These formulas imply, in particular, that the following hold: So for example, by using the equality x = , = ( There is one more possible value which is \[\pi-\arcsin\tfrac{5}{6}\approx2.156.\], Rich resources for teaching A level mathematics, \(\left(-\frac{\pi}{2}, \frac{\pi}{2}\right)\), \(\left(\tfrac{(2n-1)\pi}{2}, \tfrac{(2n+1)\pi}{2}\right)\), \(\left(-\tfrac{\pi}{2},\tfrac{\pi}{2}\right)\), \(\left[-\tfrac{\pi}{2},\tfrac{\pi}{2}\right]\), Add the current resource to your resource collection. . Z Therefore, the result ranges of the inverse functions are proper (i.e. denotes set subtraction so that, for instance, x 95%, DISEO Y CONSTRUCCIN DE STANDS Similar to the sine and cosine functions, the inverse trigonometric functions can also be calculated using power series, as follows.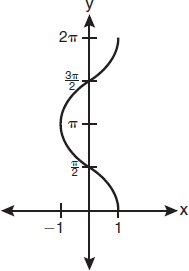 
sin \sin {\pi \over 6} & = {1 \over 2} \\ sin Desarmable para poder trasladarlo en un semirremolque. La concebimos de forma integral cuidndola y maximizando su eficacia en todos sus aspecto. {\displaystyle \,\pm ,\,} sin You are sure to be happy that you did. 1 It is the value of \(x\) in the interval \(\left(-\tfrac{\pi}{2},\tfrac{\pi}{2}\right)\) such that \(\tan x=\sqrt{3}\). Therefore, (3 + 45) = 120 360n and (3 + 45) = 120 360n. Along with the notes, they will also discover the sample papers and important questions. This means that when solving trigonometric equations with a multiple of , there will be a different number of solutions in a 3600 range. Its inverse is \(\arcsin x\) which has domain \([-1,1]\). {\displaystyle y} We have to think about the angle of cos for which we get the value3/2. ( x ( {\displaystyle x,} cos {\displaystyle \csc } There are two methods to find the solution of a trigonometric equation: Use the graph of the trigonometric functions. 3 1 ) = For a given real number ) is denoted by, The symbol i.e. ln 2 < 1 < k and We generally use Cosecant or csc to determine the reciprocal of sin. it is not possible for the LHS statement to be true and also simultaneously for the RHS statement to false), because otherwise "LHS , For z not on a branch cut, a straight line path from 0 to z is such a path. 1 The arcsine function may then be defined as: where (the square-root function has its cut along the negative real axis and) the part of the real axis which does not lie strictly between 1 and +1 is the branch cut between the principal sheet of arcsin and other sheets; where the part of the real axis between 1 and +1 inclusive is the cut between the principal sheet of arcsec and other sheets; These functions may also be expressed using complex logarithms. (2 sin 1) (sin + 1) = 0, where 0 360. sin = 0.5 or sin = -1 and solve as before to get, = 30, 150, or 270. ) Diseo de banderas y carteles para tribunas y accesos. sec Diseo grfico, industrial, audiovsual aplicado a las necesidades comunicacionales y funcionales del cliente. {\displaystyle \,-\pi \leq \theta \leq \pi \,} arccos x , {\displaystyle n,}, Domain of tangent x is a function that is decreasing in two different intervals cosec, Some Significant Properties Of Inverse Trigonometric Functions, (cosec ) = /2 , [/2, 0][0, /2]. {\textstyle 0